05 May Stress Singularities
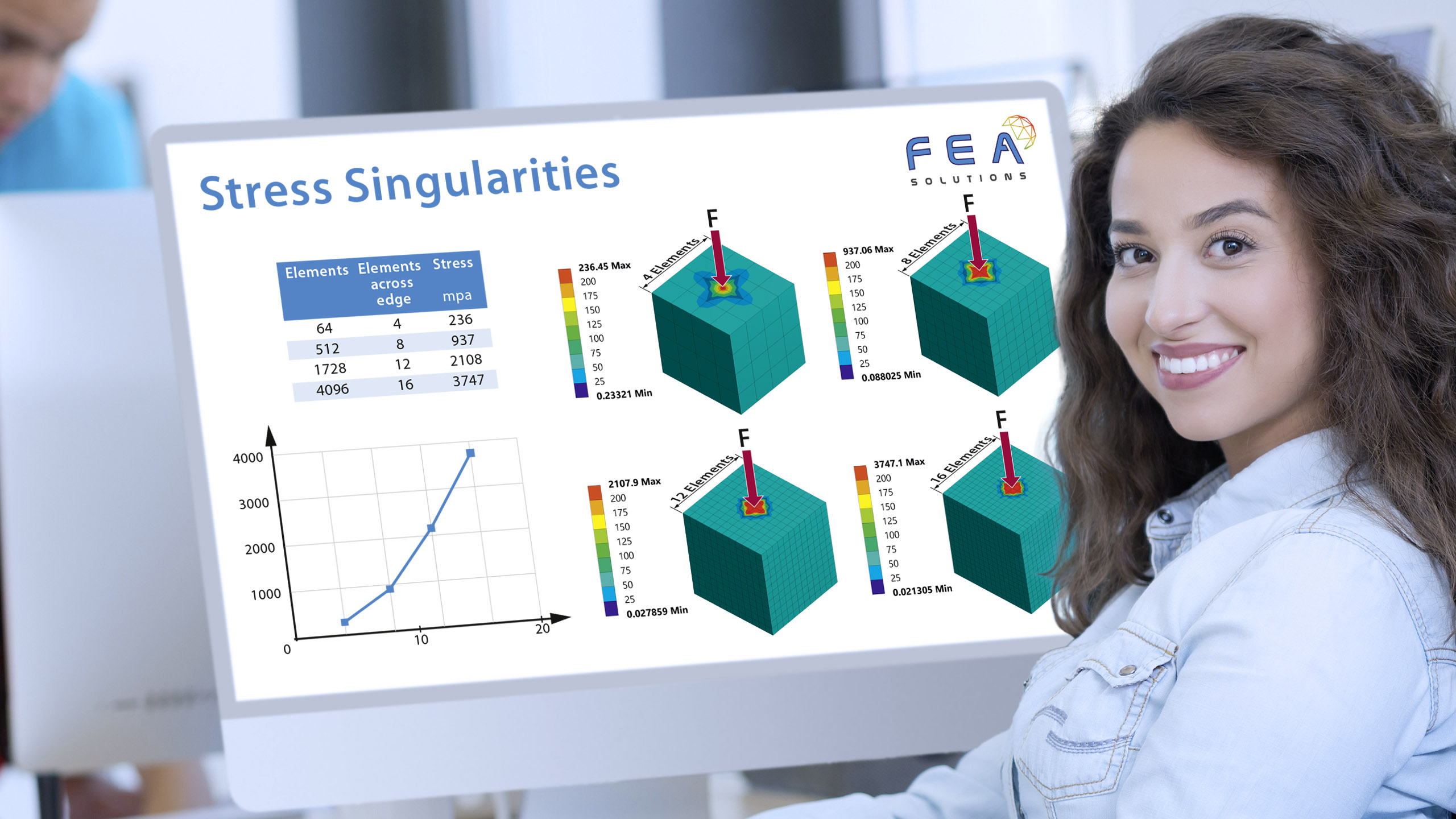
A stress singularity is a location where the stress is theoretically infinite. As stress is equal to force/area, stress singularities occur where the area is 0. There are some common places for stress singularities to occur:
- Point loads or point supports
- Sharp inside corners
A stress singularity is not the same as a stress concentration. Many geometric features may cause stress concentrations, but if the mesh in these areas is refined (mesh size reduced), the stress result will converge on a finite value. However, if the mesh is refined around a singularity, the stress will continue to increase.
Having stress singularities does not mean the stress results are incorrect elsewhere in the FEA model. The singularity will only affect the stress results at the nodes surrounding it. Further away, the stresses will be realistic, which is a consequence of St. Venant’s Principle. St. Venant’s Principle states that the effect of local disturbances to a uniform stress field remains local. Further away from the disturbance the results will not be perturbed. If the areas of interest are far away from the stress singularity, then the singularity can be ignored.
If the stress singularity is in the area of interest, there are different ways to address the issue:
- In reality, point loads or point supports do not exist. Hence, the load or constraint should be spread over an area in the FEA model, rather than applying it at one node. This will eradicate the stress singularity.
- In reality, sharp corners do not exist as there is always some radius at a corner’s location. Therefore, geometric induced singularities can be solved by introducing a fillet at that location. The stress singularity will effectively become a stress concentration. Fillet radii should be used in any case in reality to reduce the effect of stress concentrations.
- In reality, infinite stresses do not exist. The material will yield or fail at some given stress value before excessive stresses are experienced. Hence, rather than using a linear-elastic material model (https://fea-solutions.co.uk/elasticity-and-plasticity/) an elastic-plastic material model (https://fea-solutions.co.uk/stress-strain-curves-in-fea/) can be employed. An elastic-perfectly plastic model (tangent modulus is zero) will ‘cut off’ any stress increase at the singularity past the elastic limit of the material.
In all of these cases, the mesh should be refined (https://www.fea-solutions.co.uk/mesh-refinement/) regardless to ensure that the stress results do converge.
Call us today on +44 (0)1202 798991 to discuss any engineering analysis requirements you might have.
