27 Nov Optimisation of a Dynamic System
Optimisation of a Dynamic System
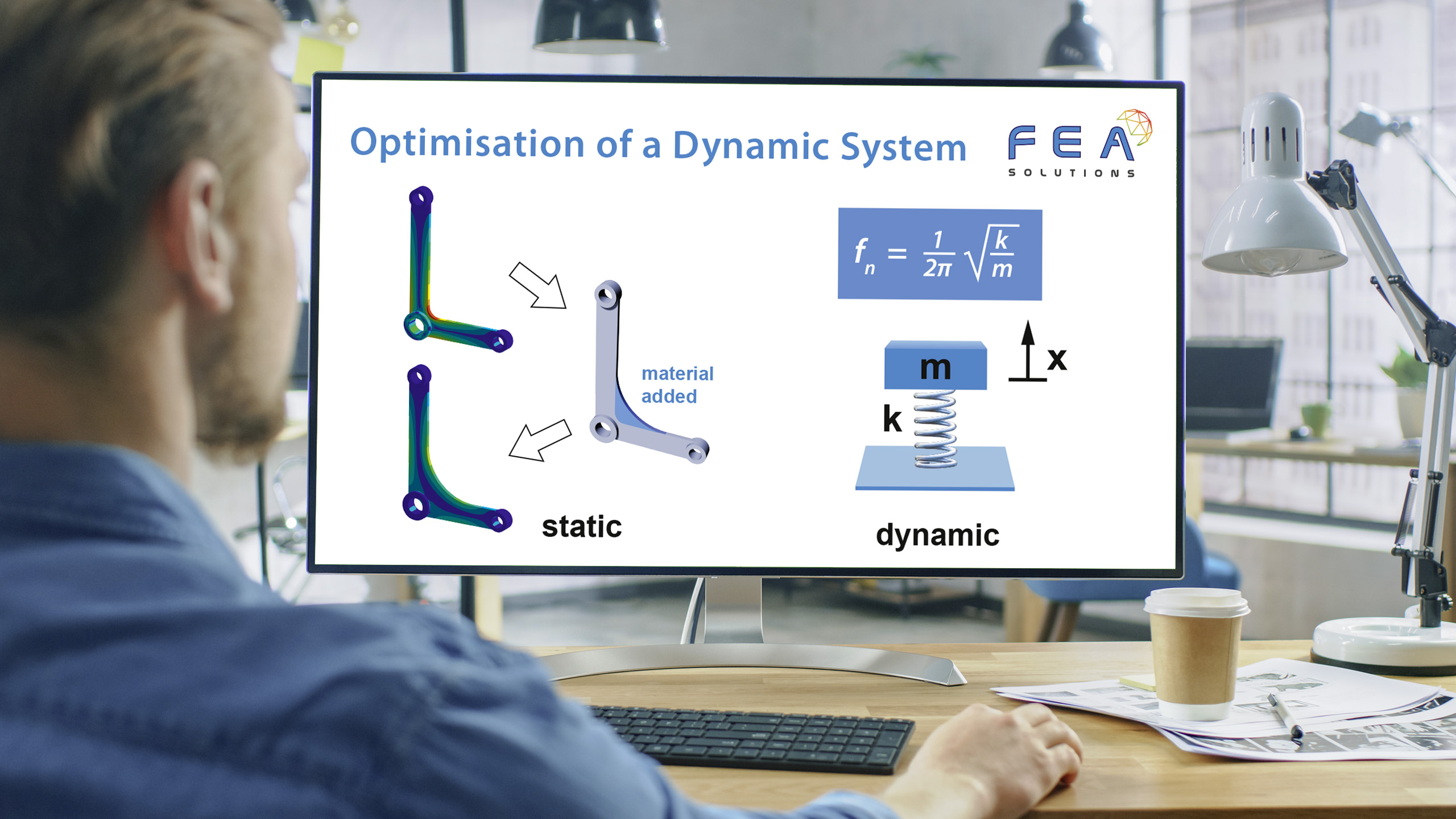
Improving the strength of a structure subjected to a static load is usually quite straight forward, by adding material to the most highly stressed areas.
Improving a structure that is subjected to dynamic loads can however be quite tricky. The difference between static and dynamic analysis is explained here: (https://fea-solutions.co.uk/static-vs-dynamic-analysis/).
A Single Degree Of Freedom (SDOF) dynamic system as shown in the image is defined by a mass m which can only move in one direction (hence single degree of freedom), attached to the base by a spring with the stiffness k. The natural frequency f of this undamped system: (https://fea-solutions.co.uk/frequency-and-period/) is proportional to:
f ~ √(k/m)
This means, when adding material to the most highly stressed areas of a dynamically loaded structure, mass and stiffness are changed at the same time, which will affect the natural frequencies and hence the dynamic response to the load, thus potentially increasing the stress rather than reducing it. This has to be kept in mind whenever comparing various options to increase the strength of a dynamic system.
Please call us today on +44 (0)1202 798991 for any engineering analysis requirements you might have.
